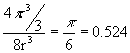The unit cell for a simple cubic cell has one sphere at each corner of the cube

2. The unit cell for a simple cubic cell has one sphere at each corner of the cube. Show that the fraction of filled space is 0.524. Assume that the spheres touch each other along every direction. Volume of a cube = a³ where a is the length of a side; volume of a sphere = 4πr³/3 where r is the radius of the sphere.

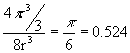
A sketch of the unit cell is shown below:

There is 1 sphere per unit cell (8 corners × 1/8 = 1) with a volume of 4πr³/3. The length of the unit cell a = 2r (since the spheres are assumed to be touching) so the volume of the cell is (2r)³ = 8r³. The fraction of filled space is